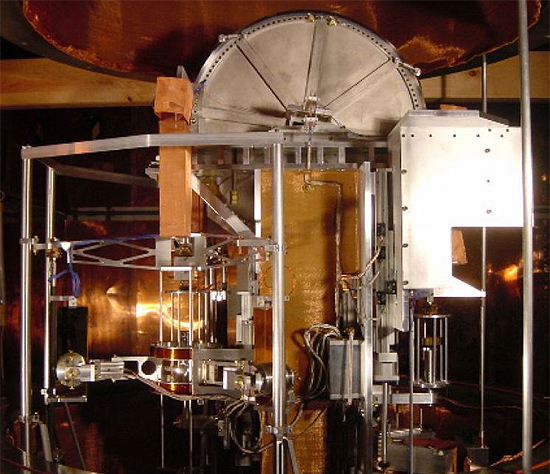
Quand j’étais plus jeune, je savais qu’un kilogramme de plume était beaucoup plus léger qu’un kilogramme de plomb, puis j’ai reconnu mon erreur, Maintenant pour connaitre le poids exact d’un Kilogramme, il faudra utiliser une balance de Kibble, (Le principe de Kibble a été proposé en 1976 par B.P. Kibble.) La mesure se déroule en deux phases, l’une dite statique, l’autre dynamique.
When I was younger, I knew that a kilogram of feather was a lot lighter than a kilogram of lead, then I recognized my mistake, Now to know the exact weight of a kilogram, you have to use a scale of Kibble, (The Kibble principle was proposed in 1976 by BP Kibble.) The measurement takes place in two phases, one called static, the other dynamic.
Phase statique
Elle consiste à équilibrer, le poids d’une masse m placée dans le champ de pesanteur g par la force de Laplace qui s’exerce sur une longueur l d’un conducteur parcouru par un courant I et placé dans un champ d’induction magnétique homogène B. Moyennant une hypothèse de configuration géométrique parfaite, l’équilibre de la balance se traduit par l’équation mg = B.l.I
Dans cette équation, la détermination expérimentale du produit B.l reste délicate et son incertitude de mesure est bien supérieure à l’incertitude relative de 10-8 recherchée. On remédie à cette difficulté en effectuant une mesure de ce produit B.l en phase dynamique.
Phase dynamique
Cette phase consiste à déplacer à vitesse constante v, le même conducteur de longueur l dans le même champ magnétique B suivant une trajectoire verticale. La force électromotrice induite E aux bornes de ce conducteur s’écrit E = B.l.v
En éliminant le produit B.l entre ces deux équations, on arrive à m.g.v = E.I
En fait je crois que je vais continuer à utiliser ma balance de cuisine, quitte à faire une erreur de quelques milligrammes…
Static phase
It consists in balancing the weight of a mass m placed in the gravitational field g by the force of Laplace which is exerted on a length l of a conductor traversed by a current I and placed in a field of magnetic induction. homogeneous B. Using a hypothesis of perfect geometrical configuration, the equilibrium of the balance results in the equation mg = BlI
In this equation, the experimental determination of the B.l product remains delicate and its measurement uncertainty is much higher than the relative uncertainty of 10-8 sought. This difficulty is remedied by performing a measurement of this B.l product in dynamic phase.
Dynamic phase
This phase consists of moving at constant speed v, the same conductor of length l in the same magnetic field B following a vertical trajectory. The electromotive force induced at the terminals of this conductor is written E = B.l.v.
By eliminating the product B.l between these two equations, we arrive at m.g.v = E.I
In fact I think I will continue to use my kitchen scale, even to make a mistake of a few milligrams …